4.6 Hertz & Radiation Fields

The Discovery of Electromagnetic Waves

In 1914, Pierre Duhem (1861–1916) haughtily proclaimed the superiority of French and German thinking in understanding field lines without the mechanical scaffolding of the English. A few decades earlier, however, both the French and Germans were largely devoted to action-at-a-distance theories. Nevertheless, it was in Germany that the great breakthrough was made. Writing in 1893, Heaviside observed:

Maxwell’s inimitable theory of dielectric displacement was for long generally regarded as a speculation. There was, for many years, an almost complete dearth of interest in the unverified parts of Maxwell’s theory… Three years ago electromagnetic waves were nowhere. Shortly after, they were everywhere. This was due to a very remarkable and unexpected event, no less than the experimental discovery by Hertz… of the veritable actuality of electromagnetic waves in the ether. And it never rains but it pours; for whilst Hertz with his resonating circuit was working in Germany (where one would least expect such a discovery to be made, if one judged solely by the old German electro-dynamic theories), Lodge was doing in some respects similar work in England, in connection with the theory of lightning conductors [[i]].

Heinrich Rudolf Hertz (1857–1894) demonstrated electromagnetic waves exist in 1888 [[ii]]. He was not the first to experiment with radio waves. Contemporaries such as David Edward Hughes (1831–1900) and Oliver Lodge (1851–1940) performed similar work in parallel. What set Hertz apart, however, was his tenacity in teasing out the properties of these waves from clever use of crude instruments [[iii]].

Hertz set up spark discharge transmitters. Knowing their inductance and capacitance, he could calculate their frequency. He set up standing waves and used a loop with a tiny gap as a detector. Where the glow or arcing across the gap was strongest, the detector was at the peak or anti-node of the standing wave. Where the glow vanished, the detector was a node or null of the standing wave.

Figure 4.50: Heinrich Hertz (1857–1894) discovered radio waves and advanced electromagnetic theory [[iv]].

His ingenious experiment gave him the wavelength. Multiplying wavelength and frequency together yielded a result for the wave velocity that demonstrated electromagnetic waves moved at the speed of light, just as had been predicted by Maxwell. Through this and similar experiments, Hertz demonstrated that electromagnetic waves had the same properties as light:

...light behaves just as the electric waves here behave; but we must imagine the dimensions of everything concerned in the experiment to be reduced in the same proportions, not only the length of the waves [[v]].

This point requires emphasis. Electrical power oscillations at 60 cycles per second, radio waves at millions to billions of cycles per second, and light at a few hundred million million cycles per second are all electromagnetic waves–the same physical phenomenon, just with different frequencies and wavelengths. In recognition of Hertz’s momentous discovery, the unit of frequency, a “cycle per second,” is called “Hertz.”

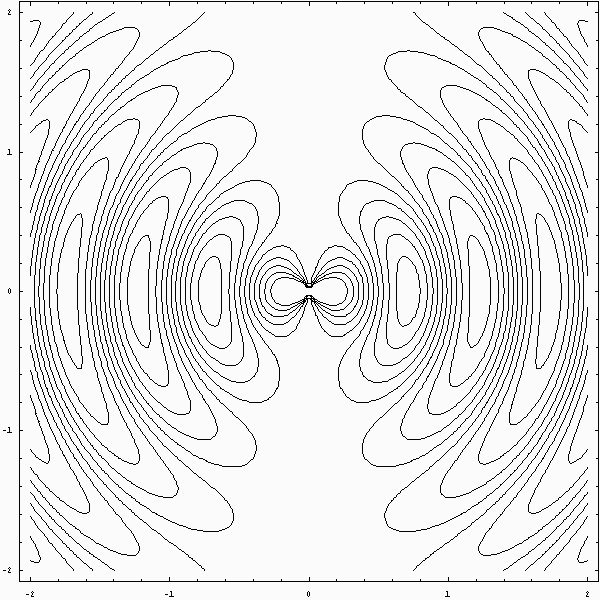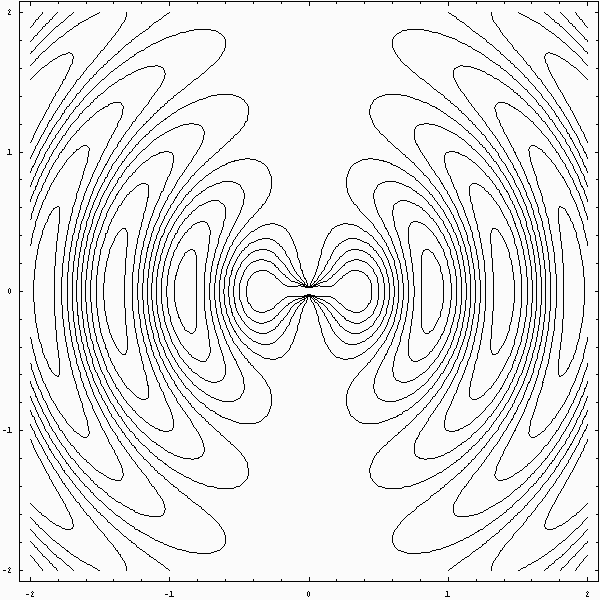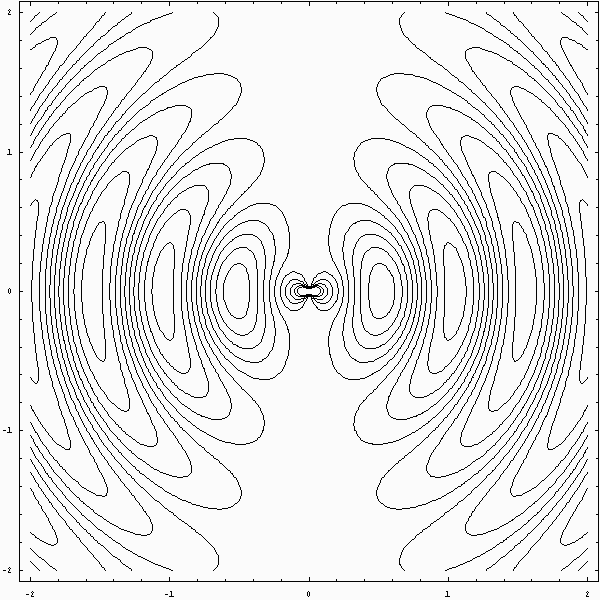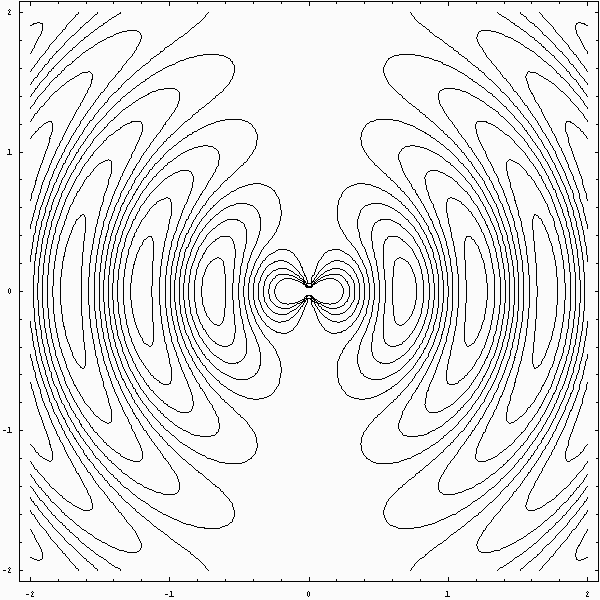
In particular, Hertz was the first to systematically explore the physics of the simplest radiating system, the dipole. A dipole may be two equal and opposite charges, as in Figure 3.18, a short current segment, as in Figure 3.21, or even a current loop, as in Figure 3.23. If the actual dipole is small compared to the wavelengths involved, the field lines follow the same “Hertzian” form first discovered and plotted by Hertz. Hertz evaluated how these field lines vary dynamically as the dipole source oscillates and radiates electromagnetic waves. Hertz lacked any sort of computer or graphing calculator to do his work yet produced the beautiful diagrams of Figure 4.51 capturing the dynamic field lines [[vi], [vii]].

Figure 4.51: Electric field lines of a harmonic electric dipole with period T at time t = 0.25 T (upper left), at time t = 0.375 T (upper right), at time t = 0. 50 T (lower left), at time t = 0.625 T (lower right). The vertical and horizontal axes are in units of wavelength.

Figure 4.51(a) shows the electric fields when the voltage is minimum, and current is maximum. In Figure 4.51(b), voltage grows and current decreases. Figure 4.51(c) shows voltage at a maximum. Figure 4.51(d) shows the voltage decreasing. Some field lines are beginning to decouple. Others are collapsing back into the dipole source. The next half period will have identical behavior except that the sense of the field lines will be reversed.

The “magic” of radiation lies in the transformation of bound, or reactive, electromagnetic fields to unbound, or radiation, fields [[viii]]. This transformation is particularly clear and striking in the electric field of an ideal point electric dipole. The harmonic dipole electric fields of Figure 4.41 correspond to an electric dipole with maximum voltage at time zero. As the dipole voltage decreases, the electric fields collapse. These field lines pinch off at about an eighth of a wavelength (λ/8) to a sixth of a wavelength (λ/6) from the dipole source. Field lines inside this pinch point collapse back into the dipole source. Field lines outside this pinch point form the closed field line loop geometry characteristic of radiation fields. Deprived of their umbilical cord back to the dipole charges, they decouple and radiate away. Figure 4.51 shows a detailed view of the field lines from a dipole of equal and opposite charges (±Q) as a radiated field is born.

Figure 4.52: Detail of electric field lines in the vicinity of an ideal point electric dipole at t = 0.1975T after dipole voltage maximum.

Sadly, the dynamic field-line plotting pioneered by Hertz, has become something of a lost art. Most contemporary texts focus on simple static field examples, if they describe field plotting at all [[ix], [x]]. Two rare exceptions that calculate the equations for the field lines of a sinusoidally oscillating dipole are Schelkunoff’s and Friis’ antenna text [[xi]] and Lorrain’s and Corson’s electromagnetics text [[xii]]. Generalizing to dipoles with an arbitrary time dependence is not difficult, and yet, I found no references in the literature. I worked it out and presented it in the second edition of my ultrawideband antenna text, if you are curious about the mathematical details [[xiii]].

Figure 4.53: The radiation approximation leaves field lines hanging from implied sources and sinks (left). Radiation fields actually form closed loops with neither sinks nor sources (right).

Interestingly enough, even many talented physicists and engineers (who should know better) fail to grasp the essential point that radiation fields must form closed loops as required by Gauss’s Law for Electricity. For an infinitesimal dipole or an electrically-small antenna, the radiation fields are often assumed to vary as (sin θ)/r, where θ is the angle with respect to the dipole axis and r is the radial distance. Figure 4.53 shows this behavior. Even the common approximation of a plane wave has this same deficiency, except that the plane-wave approximation moves the missing longitudinal fields arbitrarily far away from the region of interest.

Next time: 4.6.1 How Does Radiation Work?

Full Table of Contents [click here]

Chapter 4 Electromagnetism Comes of Age

• 4.1 Heaviside, Poynting, & Energy Flow

• 4.2 Early Models of Electromagnetism

  • 4.2.1 Ancient Views

  • 4.2.2 Fluid Theories of Electricity

• 4.3 Actions at a Distance: Fluids or Fields?

  • 4.3.1 Skin Depth and Litz Wire

  • 4.3.2 Inductive Telegraphy at a Distance

  • 4.3.3 Cross Coupling

• 4.4 Signals Along a Transmission Line

  • 4.4.1 How Do Transmission Lines Work?

  • 4.4.2 The Catt Question

• 4.5 An Introduction to Electromagnetic Models

  • 4.5.1 Potentials and Actions at a Distance

  • 4.5.2 Jefimenko & Lorentz

  • 4.5.3 A Synthesis

• 4.6 Hertz & Radiation Fields

• 4.7 How Does Radiation Work?

• 4.8 Summary & Conclusions

---

Follow Online:

You may follow me online in other places as well:

• Telegram: 𝔸𝕖𝕥𝕙𝕖𝕣𝕔𝕫𝕒𝕣'𝕤 𝔸𝕖𝕥𝕙𝕖𝕣𝕤𝕥𝕣𝕖𝕒𝕞

• Gab: @aetherczar

• Twitter: @aetherczar

• Amazon: Hans G. Schantz

• Wise of Heart Substack

• ResearchGate

• Google Scholar

• ORCID

---

References

---

[[i]]         Heaviside, Oliver, Electromagnetic Theory, vol. 1, London: The Electrician, p. 5.

[[ii]]        Hertz, Heinrich, Electric Waves, London: Macmillan and Co., 1893. Hertz is justly acclaimed for the accomplishments documented in Electric Waves. This work demonstrates Hertz’s painstaking attention to detail and his mastery of both Maxwell’s theory as well as the experimental techniques necessary to create, detect, and manipulate radio waves.

[[iii]]       Smith, Glenn S., An Introduction to Classical Electromagnetic Radiation, Cambridge: Cambridge University Press, 1997, pp. 161‑169.

[[iv]]        Porträt Heinrich Hertz, Fotografie von Robert Krewaldt, Bonn Veröffentlich bzw. erstellt von "Washington, D.C.: Underwood & Underwood", nachbearbeitet / Lizenz: Public Domain

[[v]]        Hertz, H., Electric Waves, London: MacMillan and Co., 1893, pp. 275-6.

[[vi]]        “The Forces of Electric Oscillations Treated According to Maxwell’s Theory. by Dr. H. Hertz” 1 . (1889). Nature, 39(1010), 450–452. doi:10.1038/039450b0
p. 451 .

[[vii]]       Hertz, Heinrich, Electric Waves, London: Macmillan and Co., 1893, pp. 144–145.

[[viii]]      Lekner, John, “The Birth of Radiation,” European Journal of Physics, vol. 40, 2019 025201.

[[ix]]       Smythe, William R., Static and Dynamic Electricity, 3^rd ed., New York: McGraw Hill, 1968, pp. 7–10.

[[x]]        Wangsness, Roald K., Electromagnetic Fields, 2^nd ed., New York: John Wiley & Sons, 1986, pp. 119–123.

[[xi]]       Schelkunoff, Sergei, and Harald Friis, Antennas: Theory and Practice, New York: John Wiley and Sons, 1952, pp. 126–129.

[[xii]]       Lorrain, Paul, and Dale Corson, Electromagnetic Fields and Waves, 2^nd ed., San Francisco: W.H. Freeman and Company, 1970, pp. 605–611.

[[xiii]]      Schantz, Hans, The Art and Science of Ultrawideband Antennas, 2^nd ed, Boston: Artech House, 2015, pp. 256-258.